
Now that the trees are bare you can do a little math on their branches.
Did you know that if you start at the trunk, gathering together all the branches and squeezing them tightly all the way to the top, the bundled branches will be the same circumference as the tree trunk? The tree would be one big cylinder, all the same thickness as the trunk.
This principle is Da Vinci’s Rule of Trees. More than 500 years ago Leonardo wrote: “All the branches of a tree at every stage of its height when put together are equal in thickness to the trunk.”
Intuitively we can guess this is true, but the rule is very impractical to prove by hand. A small tree could be squeezed – and sacrificed by the experiment – but a larger tree has to be measured and calculated. As physicist Christophe Eloy of University of Provence said, “If you’re looking at big trees, there’s thousands of branches, and it takes a lot of undergrads to measure it.”
Two years ago Christophe Eloy proved Da Vinci’s Rule of Trees and the reason why it occurs by designing intricately branched trees on a computer and putting them through a virtual wind tunnel.
The designs, like the trees, were branching fractals repeated over and over. With each design Eloy varied the thickness of the branches and subjected them to virtual wind forces that broke them. Invariably when he found a combination that withstood the wind, it matched Da Vinci’s rule. This not only proved the rule but showed that wind is a reason for the rule’s existence. His findings were published in the journal Physical Review Letters in 2011.
Of course Eloy’s proof involved math and physics. Here’s his diagram of the fractal thickness and one of his computed trees.
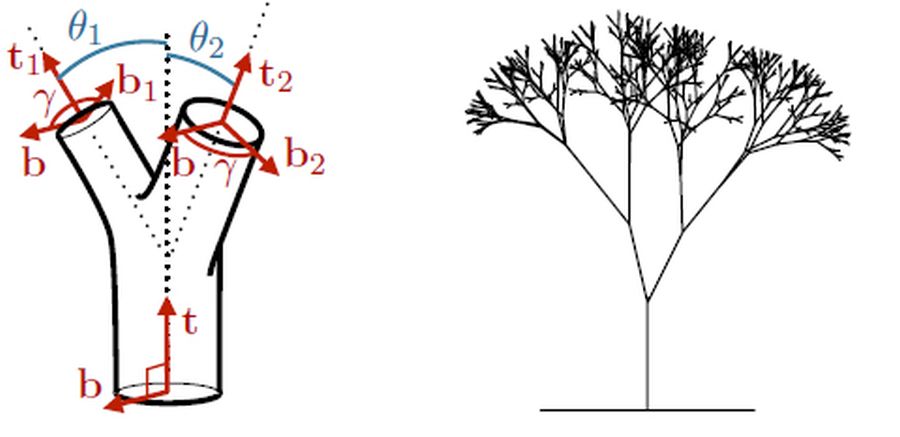
But don’t take my word for it. Read more about Christophe Eloy’s study and Da Vinci’s Rule of Trees at this link in Inside Science.
(photo of a bare tree at sunset from Wikimedia Commons. Tree diagram courtesy Christophe Eloy, University of Provence linked from Inside Science. Click on each image to see its original.)








